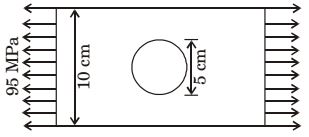
1. A large uniform plate containing a rivet hole is subjected to uniform uniaxial tension of 95 MPa. The maximum stress in the plate is
   
   
   

1. 1. 100 MPa
      

   
   
   

   2. 285 MPa
      

   
   
   

   3. 190 MPa
      

   
   
   

   4. Indetermine

   
   
   

---

1. View Hint View Answer Discuss in Forum

   
   

   σ =	P
   	A

   
   P = σ A = 95 × 10⁶ × 10 × 10^–2 t
   

   σ =	P	=	95 × 106 × 10 × 10–2 t	= 190 MPa
   	A		(10 × 10-2 - 5 × 10–2)t

   Correct Option: B

   
   

   σ =	P
   	A

   
   P = σ A = 95 × 10⁶ × 10 × 10^–2 t
   

   σ =	P	=	95 × 106 × 10 × 10–2 t	= 190 MPa
   	A		(10 × 10-2 - 5 × 10–2)t

---

2. A rod of length 20 mm is stretched to make a rod of length 40 mm. Subsequently, it is compressed to make a rod of final length 10 mm. Consider the longitudinal tensile strain as positive and compressive strain as negative. The total true longitudinal strain in the rod is
   

1. 1. –0.5
      

   
   
   

   2. –0.69
      

   
   
   

   3. –0.75
      

   
   
   

   4. –1.0

   
   
   

---

1. View Hint View Answer Discuss in Forum

   Volume remain same L₁ = 20 mm, L₂ = 40 mm, L₃ = 10mm
   A₁ L₁ = A₂ L₂ = A₃ L₃
   

   	A1	=	L3
   	A3		L1

   
   

   True strain = ln		A1		= ln		L3		= -0.693
   		A3				L1

   
   

   Correct Option: B

   Volume remain same L₁ = 20 mm, L₂ = 40 mm, L₃ = 10mm
   A₁ L₁ = A₂ L₂ = A₃ L₃
   

   	A1	=	L3
   	A3		L1

   
   

   True strain = ln		A1		= ln		L3		= -0.693
   		A3				L1

   
   

---

---

3. An elastic body is subjected to a tensile stress X in a particular direction and a compressive stress Y in its perpendicular direction. X and Y are unequal in magnitude. On the plane of maximum shear stress in the body there will be
   

1. 1. no normal stress
      

   
   
   

   2. also the maximum normal stress
      

   
   
   

   3. the minimum normal stress
      

   
   
   

   4. both normal stress and shear stress

   
   
   

---

1. View Hint View Answer Discuss in Forum

   NA

   Correct Option: C

   NA

---

4. The three-dimensional state of stress at a point is given by
   
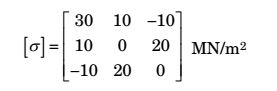
   The shear stress on the x-face in y-direction at the same point is then equal to
   

1. 1. zero MN/m²
      
      

   
   
   

   2. -10 MN/m²
      

   
   
   

   3. 10 MN/m²
      

   
   
   

   4. 20 MN/m²
      

   
   
   

---

1. View Hint View Answer Discuss in Forum

   

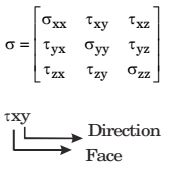
   Correct Option: C

   

---

---

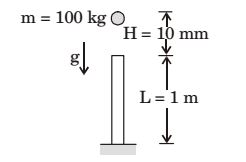
5. A point mass of 100 kg is dropped onto a massless elastic bar (cross-sectional area = 100 mm², length = 1 m, Young's modulus = 100 GPa) from a height H of 10 mm as shown (Figure is not to scale). If g = 10 m/s², the maximum compression of the elastic bar is ______ mm.
   

1. 1. 151.7 mm

   
   
   

   2. 15.17 mm

   
   
   

   3. 1.517 mm

   
   
   

   4. 11.517 mm

   
   
   

---

1. View Hint View Answer Discuss in Forum

   m = 100 kg , h = 10 mm, L = 1m
   E = 100 GPa, g = 10m/s² A = 100 mm²
   

   δ =	WL		1 + √	2EAh
   	AE			wL

   
   

   δ =	100 × 10 × 1	[ 1 + √1 + { (2 × 100 × 109 × 100 × 10-6 × 0.01) / 1000 } ]
   	100 × 10-6 × 100 × 109

   
   δ = 1.517 mm
   

   Correct Option: C

   m = 100 kg , h = 10 mm, L = 1m
   E = 100 GPa, g = 10m/s² A = 100 mm²
   

   δ =	WL		1 + √	2EAh
   	AE			wL

   
   

   δ =	100 × 10 × 1	[ 1 + √1 + { (2 × 100 × 109 × 100 × 10-6 × 0.01) / 1000 } ]
   	100 × 10-6 × 100 × 109

   
   δ = 1.517 mm
   

---