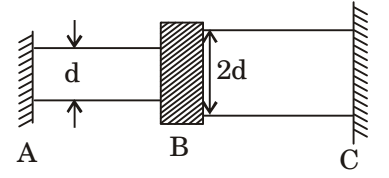
71. Two shafts AB and BC, of equal length and diameters d and 2d, are made of the same material. They are joined at B through a shaft coupling, while the ends A and C are built-in (cantilevered). A twisting moment T is applied to the coupling. If T_A and T_C represent the twisting moments at the ends A and C, respectively, then
    

1. 1. T_C = T_C

   
   
   

   2. T_C = 8T_C

   
   
   

   3. T_C = 16 T_C

   
   
   

   4. T_C = 16T_C

   
   
   

---

1. View Hint View Answer Discuss in Forum

   
   θ_A = θ_C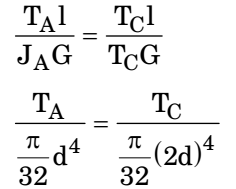
   
   T_C = 16T_A.

   Correct Option: C

   
   θ_A = θ_C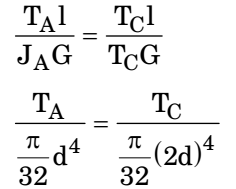
   
   T_C = 16T_A.

---

72. A torque of 1.0 Nm is transmitted through a stepped shaft as shown in figure. The torsional stiffness's of individual sections of lengths M N, NO and OP are 20 Nm/rad, 30 Nm/rad and 60 Nm/rad respectively. The angular deflection between the ends M and P of the shaft is
    

1. 1. 0.05 rad

   
   
   

   2. 0.1 rad

   
   
   

   3. 0.5 rad

   
   
   

   4. 1.0 rad

   
   
   

---

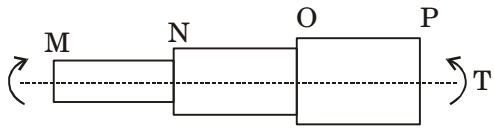
1. View Hint View Answer Discuss in Forum

   
   

   	T	= 20 Nm/rad
   	θMN

   
   T = 1 N.m
   

   θMN =	1	rad
   	20

   
   

   θNO =	1	rad
   	30

   
   

   θOP =	1	rad
   	60

   
   The part MN, NO & OP are connected in series
   θ_NP = θ_MN + θ_NO + θ_OP
   

   =	1	+	1	+	1	= 0.1 rad.
   	20		30		60

   
   θ = 0.1 rad

   Correct Option: B

   
   

   	T	= 20 Nm/rad
   	θMN

   
   T = 1 N.m
   

   θMN =	1	rad
   	20

   
   

   θNO =	1	rad
   	30

   
   

   θOP =	1	rad
   	60

   
   The part MN, NO & OP are connected in series
   θ_NP = θ_MN + θ_NO + θ_OP
   

   =	1	+	1	+	1	= 0.1 rad.
   	20		30		60

   
   θ = 0.1 rad

---

---

73. Two shafts A and B are made of the same material. The diameter of shaft B is twice that of shaft A. The ratio of power which can be transmitted by shaft A to that of shaft B is (If maximum shear stress remains the same)

1. 1. 1/2

   
   
   

   2. 1/4

   
   
   

   3. 1/8

   
   
   

   4. 1/16

   
   
   

---

1. View Hint View Answer Discuss in Forum

   d_B = 2d_A.
   τ_B = τ_A
   

   		Tr			=		Tr
   		2		A			2		B

   
   

   	TA	=	JA		rB
   	TB		JB		rA

   
   

   	2	=	1	=	1
   	24		23		8

   Correct Option: C

   d_B = 2d_A.
   τ_B = τ_A
   

   		Tr			=		Tr
   		2		A			2		B

   
   

   	TA	=	JA		rB
   	TB		JB		rA

   
   

   	2	=	1	=	1
   	24		23		8

---

74. The compound shaft shown is built-in at the two ends. It is subjected to a twisting moment T at the middle. What is the ratio of the reaction torques T₁, and T₂ at the ends?
    

1. 1. 1/16

   
   
   

   2. 1/8

   
   
   

   3. 1/4

   
   
   

   4. 1/2

   
   
   

---

1. View Hint View Answer Discuss in Forum

   
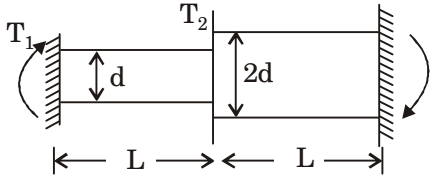
   θ ₁ = θ ₂
   

   	T1I	=	T2I
   	J1G		J2G

   
   

   	T1	=	1
   	T2		16

   Correct Option: A

   
   θ ₁ = θ ₂
   

   	T1I	=	T2I
   	J1G		J2G

   
   

   	T1	=	1
   	T2		16

---

---

75. A hollow circular shaft of inner radius 10 mm outer radius 20 mm and length 1 m is to be used as a torsional spring. If the shear modulus of the material of the shaft is 150 GPa, the torsional stiffness of the shaft (in kN-m/rad) is ______ (correct to two decimal places).

1. 1. 35.34

   
   
   

   2. 33.34

   
   
   

   3. 34.34

   
   
   

   4. 32.34

   
   
   

---

1. View Hint View Answer Discuss in Forum

   35.34 KN- m/rad
   G = 1504 Pa
   

   	T	=	GJ
   	θ		I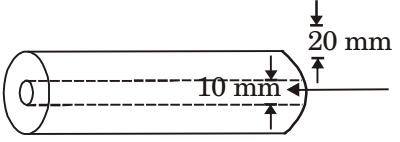

   
   
   

   =	I	=	150 × 103	×	π	× (0.044 - 0.024)
   	θ		1		32

   
   

   	I	= 35.34
   	θ

   Correct Option: A

   35.34 KN- m/rad
   G = 1504 Pa
   

   	T	=	GJ
   	θ		I

   
   
   

   =	I	=	150 × 103	×	π	× (0.044 - 0.024)
   	θ		1		32

   
   

   	I	= 35.34
   	θ

---