Any number between 100 and 1000 may be written as 100m + 10n + K where 0 < m <
9, 0 < n < 9 and 0 < K < 9.
∴  (100m + 10n + K) – (m + n + K) = 99m + 9n = 9 (11m + n) = multiplie of 9.
Hence divisible by 9.

Correct Option: B

Any number between 100 and 1000 may be written as 100m + 10n + K where 0 < m <
9, 0 < n < 9 and 0 < K < 9.
∴  (100m + 10n + K) – (m + n + K) = 99m + 9n = 9 (11m + n) = multiplie of 9.
Hence divisible by 9.

We proceed to find the number that is least as mentioned below.

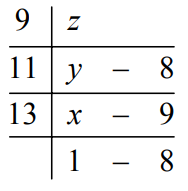
x = 13 × 1 + 8 = 21
y = 11x + 9 = 11 × 21 + 9 = 231 + 9 = 240
z = 9y + 8 = 9 × 240 = 8 = 2160 + 8 = 2168.
Now, divide 2168 by 13, 11 and 9.

Hence, the remainders are 10, 1 and 6 respectively.
Remark : To determine the least number, we have taken the last quotient as 1.

Correct Option: A

We proceed to find the number that is least as mentioned below.

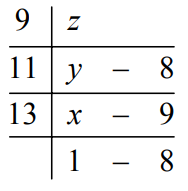
x = 13 × 1 + 8 = 21
y = 11x + 9 = 11 × 21 + 9 = 231 + 9 = 240
z = 9y + 8 = 9 × 240 = 8 = 2160 + 8 = 2168.
Now, divide 2168 by 13, 11 and 9.

Hence, the remainders are 10, 1 and 6 respectively.
Remark : To determine the least number, we have taken the last quotient as 1.

It is to be noted that 88= 8 × 11
Here, d₁ = 8, d₂ = 11, r₁ = 3, r₂ = 7.
Where d₁, d₂ are divisors and r₁ and r₂ are respective remainders.
∴  Required remainder = d₁r₂ + r₁
= 8 × 7 + 3 = 56 + 3 = 59

Correct Option: C

It is to be noted that 88= 8 × 11
Here, d₁ = 8, d₂ = 11, r₁ = 3, r₂ = 7.
Where d₁, d₂ are divisors and r₁ and r₂ are respective remainders.
∴  Required remainder = d₁r₂ + r₁
= 8 × 7 + 3 = 56 + 3 = 59

We break each base number into prime factors.
Now, 25¹²
= (5 × 5)¹² = 5¹² × 5¹²
10⁷ = (2 × 5)⁷ = 2⁷ × 5⁷
and , 14⁷ = (2 × 7)⁷ = 2⁷ × 7⁷
∴  25¹² × 10⁷ × 14⁷ = 5¹² × 5¹² × 2⁷ × 5⁷ × 2⁷ × 7⁷
= 5^{12 + 12 + 7} × 2⁷ + 7 × 7⁷ = 5³¹ × 2¹⁴ × 7⁷
∴  Number of prime factors = 31 + 14 + 7 = 52

Correct Option: B

We break each base number into prime factors.
Now, 25¹²
= (5 × 5)¹² = 5¹² × 5¹²
10⁷ = (2 × 5)⁷ = 2⁷ × 5⁷
and , 14⁷ = (2 × 7)⁷ = 2⁷ × 7⁷
∴  25¹² × 10⁷ × 14⁷ = 5¹² × 5¹² × 2⁷ × 5⁷ × 2⁷ × 7⁷
= 5^{12 + 12 + 7} × 2⁷ + 7 × 7⁷ = 5³¹ × 2¹⁴ × 7⁷
∴  Number of prime factors = 31 + 14 + 7 = 52

We break each base number into prime factors.
Now, 307= (2 × 3 × 5)⁷= 2⁷ × 3⁷ × 5⁷
22⁵ = (2 × 11)⁵ = 2⁵ × 11⁵
34¹² = (2 × 17)¹² = 2¹² × 17¹²
12⁵ = (3 × 2 × 2)⁵ = 3⁵ × 2⁵ × 2⁵
∴  30⁷ × 2² × 34¹² × 12⁵ = 2⁷ × 3⁷ × 5⁷ × 2⁵ × 11⁵ × 2¹² × 17¹² × 3⁵ × 2⁵ × 2⁵
= 2^7+5+12+5+5 × 3⁷⁺⁵ × 5⁷ × 11⁵ × 17¹²
= 2³⁴ × 3¹² × 5⁷ × 11⁵ × 17¹²
∴  The required number of prime factors
= 34 + 12 + 7 + 5 + 12 = 70

Correct Option: A

We break each base number into prime factors.
Now, 307= (2 × 3 × 5)⁷= 2⁷ × 3⁷ × 5⁷
22⁵ = (2 × 11)⁵ = 2⁵ × 11⁵
34¹² = (2 × 17)¹² = 2¹² × 17¹²
12⁵ = (3 × 2 × 2)⁵ = 3⁵ × 2⁵ × 2⁵
∴  30⁷ × 2² × 34¹² × 12⁵ = 2⁷ × 3⁷ × 5⁷ × 2⁵ × 11⁵ × 2¹² × 17¹² × 3⁵ × 2⁵ × 2⁵
= 2^7+5+12+5+5 × 3⁷⁺⁵ × 5⁷ × 11⁵ × 17¹²
= 2³⁴ × 3¹² × 5⁷ × 11⁵ × 17¹²
∴  The required number of prime factors
= 34 + 12 + 7 + 5 + 12 = 70