Ratio, Proportion
- Two alloys are both made up of copper and tin. The ratio of copper and tin in the first alloy is 1 : 3 and in the second alloy is 2 : 5. In what ratio should the two alloys be mixed to obtain a new alloy in which the ratio of tin and copper be 8 : 3 ?
-
View Hint View Answer Discuss in Forum
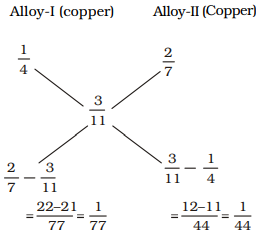
Required ratio = 1 : 1 = 4 : 7 77 44 Correct Option: B

Required ratio = 1 : 1 = 4 : 7 77 44
- A mixture contains alcohol and water in the ratio 4 : 3. If 5 litres of water is added to the mixture, the ratio becomes 4 : 5. The quantity of alcohol in the given mixture is
-
View Hint View Answer Discuss in Forum
In original mixture,
Alcohol = 4x litres
Water = 3x litres
On adding 5 litres of water,4x = 4 3x + 5 5
⇒ 20x = 12x + 20
⇒ 8x = 20⇒ x = 20 = 5 8 2
⇒ Quantity of alcohol= 4x = 4 × 5 2
= 10 litresCorrect Option: D
In original mixture,
Alcohol = 4x litres
Water = 3x litres
On adding 5 litres of water,4x = 4 3x + 5 5
⇒ 20x = 12x + 20
⇒ 8x = 20⇒ x = 20 = 5 8 2
⇒ Quantity of alcohol= 4x = 4 × 5 2
= 10 litres
- In two alloys A and B, the ratio of zinc to tin is 5 : 2 and 3 : 4 respectively. Seven kg of the alloy A and 21 kg of the alloy B are mixed together to form a new alloy. What will be the ratio of zinc and tin in the new alloy ?
-
View Hint View Answer Discuss in Forum
In 7 kg of alloy A,
Zinc = 5 kg, Tin = 2 kg
In 21 kg of alloy BZinc = 21 × 3 = 9 kg 7 Tin = 21 × 4 = 12 kg 7
∴ Required ratio
= (5 + 9) : (2 + 12) = 14 : 14
or 1 : 1Correct Option: D
In 7 kg of alloy A,
Zinc = 5 kg, Tin = 2 kg
In 21 kg of alloy BZinc = 21 × 3 = 9 kg 7 Tin = 21 × 4 = 12 kg 7
∴ Required ratio
= (5 + 9) : (2 + 12) = 14 : 14
or 1 : 1
- Zinc and copper are in the ratio 5 : 3 in 400 gm of an alloy. How much of copper (in grams) should be added to make the ratio 5 : 4?
-
View Hint View Answer Discuss in Forum
In 400 gm of alloy,
Zinc = 5 × 400 = 250 gm. 8 Copper = 3 × 400 = 150 gm. 8
If x gm of copper be mixed, then250 = 5 150 + x 4
⇒ 750 + 5x = 1000
⇒ 5x = 1000 – 750 = 250
⇒ x = 50 gmCorrect Option: A
In 400 gm of alloy,
Zinc = 5 × 400 = 250 gm. 8 Copper = 3 × 400 = 150 gm. 8
If x gm of copper be mixed, then250 = 5 150 + x 4
⇒ 750 + 5x = 1000
⇒ 5x = 1000 – 750 = 250
⇒ x = 50 gm
- Two vessels A and B contain milk and water mixed in the ratio 8 : 5 and 5 : 2 respectively. The ratio in which these two mixtures be mixed to get a new mixture containing
69 3 % milk is: 13
-
View Hint View Answer Discuss in Forum
Milk in the resulting mixture = 9 13 
= 65 − 63 = 2 7 × 13 7 × 13 ∴ Required ratio = 2 : 1 7 × 13 13
= 2 : 7Correct Option: D
Milk in the resulting mixture = 9 13 
= 65 − 63 = 2 7 × 13 7 × 13 ∴ Required ratio = 2 : 1 7 × 13 13
= 2 : 7

