Ratio, Proportion
- The ratio of income of two persons is 5 : 3 and that of their expenditures is 9 : 5. Find the income of each person, if they save ₹ 1,300 and ₹ 900 respectively.
-
View Hint View Answer Discuss in Forum
Let income of two persons be 5x and 3x.
and their expenses be 9y and 5y respectively.
Then, 5x – 9y = 1300 ..(i)
and 3x – 5y = 900 ..(ii)
By 9 × (ii) – 5 × (i), we get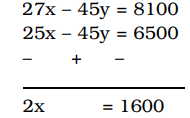
⇒ x = 800
Now, income of first person
= 5x = 5 × 800 = ₹ 4000
and that of second person
= 3x = 3 × 800 = ₹ 2400Correct Option: A
Let income of two persons be 5x and 3x.
and their expenses be 9y and 5y respectively.
Then, 5x – 9y = 1300 ..(i)
and 3x – 5y = 900 ..(ii)
By 9 × (ii) – 5 × (i), we get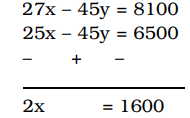
⇒ x = 800
Now, income of first person
= 5x = 5 × 800 = ₹ 4000
and that of second person
= 3x = 3 × 800 = ₹ 2400
- A and B have monthly incomes in the ratio 5 : 6 and monthly expenditures in the ratio 3 : 4. If they save ₹ 1800 and ₹ 1600 respectively, find the monthly income of B :
-
View Hint View Answer Discuss in Forum
Given
Monthly income of A = 5 Monthly income of B 6
∴ Monthly income of A = 5x
and that of B = 6x (x is a constant)
According to the question5x − 1800 = 3 6x − 1600 4
20x – 7200 = 18x – 4800
2x = 2400
∴ x = 1200
∴ Monthly income of B
= 1200 × 6 = ₹ 7200Correct Option: D
Given
Monthly income of A = 5 Monthly income of B 6
∴ Monthly income of A = 5x
and that of B = 6x (x is a constant)
According to the question5x − 1800 = 3 6x − 1600 4
20x – 7200 = 18x – 4800
2x = 2400
∴ x = 1200
∴ Monthly income of B
= 1200 × 6 = ₹ 7200
- Between two consecutive years my income are in the ratio of 2 : 3 and expenses in the ratio 5 : 9. If my income in the second year is ₹ 45000 and my expenses in the first year is ₹ 25000 my total savings for the two years is :
-
View Hint View Answer Discuss in Forum
Income in the second year
= ₹ 45000
Income in the first year
= ₹ 30000
Expense in the first year
= ₹ 25000
Expense in the second year
= ₹ 45000
∴ Total saving
= 75000 – 70000 = ₹ 5000Correct Option: D
Income in the second year
= ₹ 45000
Income in the first year
= ₹ 30000
Expense in the first year
= ₹ 25000
Expense in the second year
= ₹ 45000
∴ Total saving
= 75000 – 70000 = ₹ 5000
- The income of A, B and C are in the ratio 3 : 7 : 4 and their expenses in the ratio 4 : 3 : 5. If A saves ₹ 300 out of an income of ₹ 2,400, the savings of B and C are :
-
View Hint View Answer Discuss in Forum
Let the income of A, B and C be ₹ 3x, ₹ 7x and ₹ 4x respectively and their expenses be ₹ 4y, ₹ 3y and ₹ 5y respectively.
∴ 3x = 2400
⇒ x = 800
∴ 4y = 2400 – 300 = 2100
⇒ y = 525
∴ B’s saving = (7x – 3y)
= ₹ (7 × 800 – 3 × 525)
= ₹ (5600 – 1575)
= ₹ 4025
and C’s savings = ₹ (4x – 5y)
= ₹ (3200 – 2625) = ₹ 575Correct Option: A
Let the income of A, B and C be ₹ 3x, ₹ 7x and ₹ 4x respectively and their expenses be ₹ 4y, ₹ 3y and ₹ 5y respectively.
∴ 3x = 2400
⇒ x = 800
∴ 4y = 2400 – 300 = 2100
⇒ y = 525
∴ B’s saving = (7x – 3y)
= ₹ (7 × 800 – 3 × 525)
= ₹ (5600 – 1575)
= ₹ 4025
and C’s savings = ₹ (4x – 5y)
= ₹ (3200 – 2625) = ₹ 575
- There are three bottles of mixture of syrup and water of ratios 2 : 3, 3 : 4 and 7 : 5. 10 litres of the first and 21 litres of the second bottles are taken. How much quantity from third bottle is to be taken so that final mixture from three bottles will be of ratios 1 : 1.
-
View Hint View Answer Discuss in Forum
NA
Correct Option: D
NA

