Ratio, Proportion
- In two blends of mixed tea, the ratios of Darjeeling and Assam tea are 4 : 7 and 2 : 5. The ratio in which these two blends should be mixed to get the ratio of Darjeeling and Assam tea in the new mixture as 6 : 13 is
-
View Hint View Answer Discuss in Forum
By the rule of alligation,
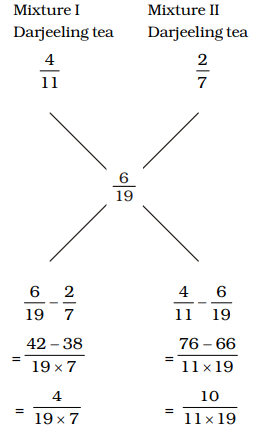
∴ Required ratio = 4 : 10 19 × 7 11 × 19 = 4 : 10 7 11
= 44 : 70 = 22 : 35Correct Option: A
By the rule of alligation,

∴ Required ratio = 4 : 10 19 × 7 11 × 19 = 4 : 10 7 11
= 44 : 70 = 22 : 35
- In a mixture of three varieties of tea, the ratio of their weights is 4 : 5 : 8. If 5 kg tea of the first variety, 10 kg tea of the second variety and some quantity of tea of the third variety are added to the mixture, the ratio of the weights of three varieties of tea becomes as 5 : 7 : 9. In the final mixture, the quantity (in kg) of the third variety of tea was
-
View Hint View Answer Discuss in Forum
Let quantity of first variety of tea = 4x kg.
Quantity of second variety of tea = 5x kg.
Quantity of third variety of tea = 8x kg.
Let y kg of third variety of tea be mixed.
∴ Resultant ratio = (4x + 5) : (5x + 10) : (8x + y)∴ 4x + 5 = 5 5x + 10 7
⇒ 28x + 35 = 25 x + 50
⇒ 28x – 25x = 50 – 35⇒ 3x = 15 ⇒ x = 15 = 5 3 ∴ 5x + 10 = 7 8x + y 9 ⇒ 5 × 5 + 10 = 7 8 × 5 + y 9 ⇒ 35 = 7 40 + y 9
⇒ 40 + y = 9 × 5
⇒ y = 45 – 40 = 5 kg.
∴ Required quantity of third variety of tea
= 8x + y = 8 × 5 + 5 = 45 kg.Correct Option: B
Let quantity of first variety of tea = 4x kg.
Quantity of second variety of tea = 5x kg.
Quantity of third variety of tea = 8x kg.
Let y kg of third variety of tea be mixed.
∴ Resultant ratio = (4x + 5) : (5x + 10) : (8x + y)∴ 4x + 5 = 5 5x + 10 7
⇒ 28x + 35 = 25 x + 50
⇒ 28x – 25x = 50 – 35⇒ 3x = 15 ⇒ x = 15 = 5 3 ∴ 5x + 10 = 7 8x + y 9 ⇒ 5 × 5 + 10 = 7 8 × 5 + y 9 ⇒ 35 = 7 40 + y 9
⇒ 40 + y = 9 × 5
⇒ y = 45 – 40 = 5 kg.
∴ Required quantity of third variety of tea
= 8x + y = 8 × 5 + 5 = 45 kg.
- The ratio of the volume of water and glycerine in 240cc of a mixture is 1 : 3. The quantity of water (in cc) that should be added to the mixture so that the new ratio of the volumes of water and glycerine becomes 2:3 is
-
View Hint View Answer Discuss in Forum
In the original mixture, water = 60 cc
Glycerine = 180 cc
Let x cc of water be mixed.∴ 60 + x = 2 180 3
⇒ 180 + 3x = 360
⇒ 3x = 360 – 180 = 180∴ x = 180 = 60 cc 3 Correct Option: B
In the original mixture, water = 60 cc
Glycerine = 180 cc
Let x cc of water be mixed.∴ 60 + x = 2 180 3
⇒ 180 + 3x = 360
⇒ 3x = 360 – 180 = 180∴ x = 180 = 60 cc 3
- Vessels A and B contain mixtures of milk and water in the ratios 4 : 5 and 5 : 1 respectively. In what ratio should quantities of mixture be taken from A and B to form a mixture in which milk to water is in the ratio 5 : 4?
-
View Hint View Answer Discuss in Forum
First of all we write the fraction of milk present in three mixtures.
In A; 4 and In B; 5 9 6 In combination of A and B; 5 9
From alligation rule,
= 5 : 1 18 9 ⇒ 5 : 2 ⇒ 5 : 2 18 18
So, ratio of
A : B = 5 : 2Correct Option: C
First of all we write the fraction of milk present in three mixtures.
In A; 4 and In B; 5 9 6 In combination of A and B; 5 9
From alligation rule,
= 5 : 1 18 9 ⇒ 5 : 2 ⇒ 5 : 2 18 18
So, ratio of
A : B = 5 : 2
- The milk and water in a mixture are in the ratio 7 : 5. When 15 litres of water are added to it, the ratio of milk and water in the new mixture becomes 7 : 8. The total quantity of water in the new mixture is
-
View Hint View Answer Discuss in Forum
Let the quantity of milk in the
mixture = 7x litres and that of
water = 5x litres.
According to the question,7x = 7 5x + 15 8
⇒ 56x = 35x + 105
⇒ 56 x – 35 x = 105
⇒ 21x = 105⇒ x = 105 = 5 21
∴ Required quantity of water
= (5x + 15) litres
= 5 × 5 + 15 = 40 litresCorrect Option: B
Let the quantity of milk in the
mixture = 7x litres and that of
water = 5x litres.
According to the question,7x = 7 5x + 15 8
⇒ 56x = 35x + 105
⇒ 56 x – 35 x = 105
⇒ 21x = 105⇒ x = 105 = 5 21
∴ Required quantity of water
= (5x + 15) litres
= 5 × 5 + 15 = 40 litres

