Ratio, Proportion

Ratio, Proportion

Easy Questions
Moderate Questions
Difficult Questions
Ratio, Proportion Tutorial

Aptitude

Simplification
Number System
Fractions
Elementary Algebra
LCM and HCF
Approximation
Unitary Method
Linear Equation
Quadratic Equation
Discount
Average
Surds and Indices
Percentage
Square root and cube root
Order of Magnitude
Work and Wages
Odd Man Out and Series
Algebra
Profit and Loss
Stocks and Shares
True Discount
Ratio, Proportion
Partnership
Alligation or Mixture
Time and Work
Pipes and Cistern
Speed, Time and Distance
Problem on Trains
Height and Distance
Banker's Discount
Boats and Streams
Races and games
Problems on Ages
Clocks and Calendars
Simple interest
Compound Interest
Sets and Functions
Area and Perimeter
Volume and Surface Area of Solid Figures
Sequences and Series
Plane Geometry
Logarithm
Probability
Permutation and Combination
Statistics
Mensuration
Trigonometry
Aptitude miscellaneous
  1. A’s income is Rs. 140 more than B’s income and C’s income is Rs. 80 more than D’s. If the ratio of A’s and C’s incomes is 2 : 3 and the ratio of B’s and D’s incomes is 1 : 2, then the incomes of A, B, C and D are respectively








  1. View Hint View Answer Discuss in Forum

    Let A‘s income be Rs. 2x.
    ∴  B’s income = Rs. (2x – 140)
    C’s income = Rs. 3x
    ∴  D’s income = Rs. (3x – 80)
    According to the question,
    B : D = 1 : 2
    ∴  2 (2x – 140) = 3x – 80
    ⇒  4x – 280 = 3x – 80
    ⇒  4x – 3x = 280 – 80
    ⇒  x = Rs. 200
    ∴  A’s income = Rs. (2 × 200) =
    Rs. 400
    B’s income = Rs. (400 – 140)
    = Rs. 260
    C’s income = Rs. (3 × 200)
    = Rs. 600
    D’s income = Rs. (600 – 80)
    = Rs. 520

    Correct Option: C

    Let A‘s income be Rs. 2x.
    ∴  B’s income = Rs. (2x – 140)
    C’s income = Rs. 3x
    ∴  D’s income = Rs. (3x – 80)
    According to the question,
    B : D = 1 : 2
    ∴  2 (2x – 140) = 3x – 80
    ⇒  4x – 280 = 3x – 80
    ⇒  4x – 3x = 280 – 80
    ⇒  x = Rs. 200
    ∴  A’s income = Rs. (2 × 200) =
    Rs. 400
    B’s income = Rs. (400 – 140)
    = Rs. 260
    C’s income = Rs. (3 × 200)
    = Rs. 600
    D’s income = Rs. (600 – 80)
    = Rs. 520

  1. A man spends a part of his monthly income and saves the rest. The ratio of his expenditure to the savings is 61 : 6. If his monthly income is Rs. 8710, the amount of his monthly savings is








  1. View Hint View Answer Discuss in Forum

    Expenditure : Savings
    = 61 : 6
    Sum of the terms of ratio
    = 61 + 6 = 67
    Total monthly salary
    = Rs. 8710
    ∴  Monthly savings

    = Rs.
    6
    		 × 8710
    67

    = Rs. 780

    Correct Option: D

    Expenditure : Savings
    = 61 : 6
    Sum of the terms of ratio
    = 61 + 6 = 67
    Total monthly salary
    = Rs. 8710
    ∴  Monthly savings

    = Rs.
    6
    		 × 8710
    67

    = Rs. 780

  1. A and B have their monthly incomes in the ratio 8 : 5, while their monthly expenditures are in the ratio 5 : 3. If they have saved Rs. 12,000 and Rs. 10,000 monthly respectively, then the difference in their monthly incomes is








  1. View Hint View Answer Discuss in Forum

    A’s monthly income = Rs. 8x
    A’s monthly expenditure= Rs. 5y
    B’s monthly income = Rs. 5x
    B’s monthly expenditure= Rs. 3y
    According to the question,
    8x – 5y = 12000      ....(i)
    5x – 3y = 10000       ....(ii)
    By equation (i) × 3 – (ii) × 5,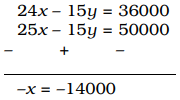

    ⇒  x = 14000
    Difference between monthly incomes of A and B = 8x – 5x
    = Rs. 3x = Rs. (3 × 14000)
    = Rs. 42000

    Correct Option: B

    A’s monthly income = Rs. 8x
    A’s monthly expenditure= Rs. 5y
    B’s monthly income = Rs. 5x
    B’s monthly expenditure= Rs. 3y
    According to the question,
    8x – 5y = 12000      ....(i)
    5x – 3y = 10000       ....(ii)
    By equation (i) × 3 – (ii) × 5,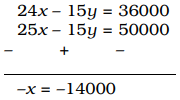

    ⇒  x = 14000
    Difference between monthly incomes of A and B = 8x – 5x
    = Rs. 3x = Rs. (3 × 14000)
    = Rs. 42000

  1. The incomes of A and B are in the ratio 3 : 2 and their expenditures are in the ratio 5 : 3. If each saves Rs. 1000, then A’s income is








  1. View Hint View Answer Discuss in Forum

    Let incomes of A and B be Rs. 3x and Rs. 2x respectively. Let the expenditures of A and B be Rs. 5y and Rs. 3y respectively.
    According to the question,
    3x – 5y = Rs. 1000 .... (i)
    2x – 3y = Rs. 1000 .... (ii)
    By equation (i) × 2 – (ii) × 3,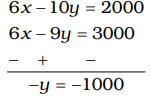

    ∴  y = 1000
    From equation (i),
    3x – 5 × 1000 = 1000
    ⇒  3x = 1000 + 5000 = Rs. 6000
    = A’s income

    Correct Option: A

    Let incomes of A and B be Rs. 3x and Rs. 2x respectively. Let the expenditures of A and B be Rs. 5y and Rs. 3y respectively.
    According to the question,
    3x – 5y = Rs. 1000 .... (i)
    2x – 3y = Rs. 1000 .... (ii)
    By equation (i) × 2 – (ii) × 3,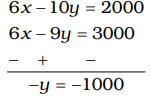

    ∴  y = 1000
    From equation (i),
    3x – 5 × 1000 = 1000
    ⇒  3x = 1000 + 5000 = Rs. 6000
    = A’s income

  1. Incomes of x and y are in the ratio 4:3. Their expenditures are in the ratio 12:7. Both save Rs. 3200 at the end of the month, then the income of x is








  1. View Hint View Answer Discuss in Forum

    x’s income = Rs. 4a
    y’s income = Rs. 3a
    x’s expenditure = Rs. 12b
    y’s expenditure = Rs. 7b
    ∴  4a – 12b = 3200
    ⇒  a – 3b = 800      ...(i)
    Again, 3a – 7b = 3200      ...(ii)
    By equation (i) × 7 – (ii) × 3,

    ⇒  a = 2000
    ⇒  x’s income = 4a
    = 4 × 2000 = Rs. 8000

    Correct Option: A

    x’s income = Rs. 4a
    y’s income = Rs. 3a
    x’s expenditure = Rs. 12b
    y’s expenditure = Rs. 7b
    ∴  4a – 12b = 3200
    ⇒  a – 3b = 800      ...(i)
    Again, 3a – 7b = 3200      ...(ii)
    By equation (i) × 7 – (ii) × 3,

    ⇒  a = 2000
    ⇒  x’s income = 4a
    = 4 × 2000 = Rs. 8000