66. If cosx = –	3	and p < x <	3π	, then the value of sin 2x will be
    	5		2

1. 1. 12
      25

   
   
   

   2. 1
      15

   
   
   

   3. 24
      25

   
   
   

   4. 5
      26

   
   
   

---

1. View Hint View Answer Discuss in Forum

   Here,
   

   cosx = –	3	and p < x <	3π
   	5		2

   
   ⇒ x lies in third quadrant, and we know that in third quadrant only tan and cot are positive.
   Consider right angled ∆ABC,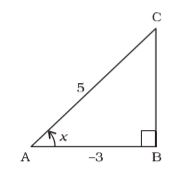
   
   Using pythagoras theorem,
   AC² = AB² + BC²
   ⇒ 5² = (–3)² + BC²
   16 = BC²
   ⇒ BC = 4
   We know that,
   sin2A = 2sinA × cosA
   ⇒ sin2x = 2 × sinx× cosx
   

   = 2 ×		- 4		×	- 3
   		5			5

   
   

   =	24
   	25

   
   ∵ Here, sinq is –ve

   Correct Option: C

   Here,
   

   cosx = –	3	and p < x <	3π
   	5		2

   
   ⇒ x lies in third quadrant, and we know that in third quadrant only tan and cot are positive.
   Consider right angled ∆ABC,
   
   Using pythagoras theorem,
   AC² = AB² + BC²
   ⇒ 5² = (–3)² + BC²
   16 = BC²
   ⇒ BC = 4
   We know that,
   sin2A = 2sinA × cosA
   ⇒ sin2x = 2 × sinx× cosx
   

   = 2 ×		- 4		×	- 3
   		5			5

   
   

   =	24
   	25

   
   ∵ Here, sinq is –ve

---

67. What is the value of tan 330° ?
    

1. 1. 1
      √3

   
   
   

   2. 0

   
   
   

   3. 1

   
   
   

   4. -	1
      	√3

   
   
   

---

1. View Hint View Answer Discuss in Forum

   tan 330° = tan(360° – 30°)
   = –tan 30°
   ∵ tan(360° - θ) - tanθ
   

   =	- 1
   	√2

   Correct Option: D

   tan 330° = tan(360° – 30°)
   = –tan 30°
   ∵ tan(360° - θ) - tanθ
   

   =	- 1
   	√2

---

---

68. The degree measure of		5π		will be
    		12

    
    

1. 1. 105°
      

   
   
   

   2. 75°
      

   
   
   

   3. 85°
      

   
   
   

   4. 110°

   
   
   

---

1. View Hint View Answer Discuss in Forum

   We know that,
   

   1R =

   180°

   =

   5π

   R

   =

   180°

   ×

   5π

   °

   = 75°

   π

   12

   π

   12

   Correct Option: B

   We know that,
   

   1R =

   180°

   =

   5π

   R

   =

   180°

   ×

   5π

   °

   = 75°

   π

   12

   π

   12

---

69. If sin (2a + 45°) = cos (30° – a), where 0° < a < 90°, then the value of a is :

1. 1. 0°

   
   
   

   2. 15°

   
   
   

   3. 45°

   
   
   

   4. 60°

   
   
   

---

1. View Hint View Answer Discuss in Forum

   sin (2a + 45°) = cos (30° – a)
   ⇒ sin (2a + 45°) = sin {90° – (30° – a) }
   ⇒ sin (2a + 45°) = sin (60° + a)
   [∵ sin (90° – θ) = cosθ]
   ⇒ 2a + 45° = 60° + a
   ⇒ 2a – a = 60° - 45°
   ⇒ a = 15°

   Correct Option: B

   sin (2a + 45°) = cos (30° – a)
   ⇒ sin (2a + 45°) = sin {90° – (30° – a) }
   ⇒ sin (2a + 45°) = sin (60° + a)
   [∵ sin (90° – θ) = cosθ]
   ⇒ 2a + 45° = 60° + a
   ⇒ 2a – a = 60° - 45°
   ⇒ a = 15°

---

---

70. The least value of tan²x + cot²x is:

1. 1. 3

   
   
   

   2. 2

   
   
   

   3. 0

   
   
   

   4. 1

   
   
   

---

1. View Hint View Answer Discuss in Forum

   The minimum value of a tan²x + b cot²x = 2√ab
   ∴ The minimum value of tan²x + cot²x = 2

   Correct Option: B

   The minimum value of a tan²x + b cot²x = 2√ab
   ∴ The minimum value of tan²x + cot²x = 2

---