26. An SCR having a turn ON time of 5 μsec, latching current of 50 mA and holding current of 40 mA is triggered by a short duration pulse and is used in the circuit shown in the figure given below. The minimum pulse width required to turn the SCR ON will be
    
    

1. 1. 251 μsec

   
   
   

   2. 150 μsec

   
   
   

   3. 100 μsec

   
   
   

   4. 5 μsec

   
   
   

---

1. View Hint View Answer Discuss in Forum

   
   

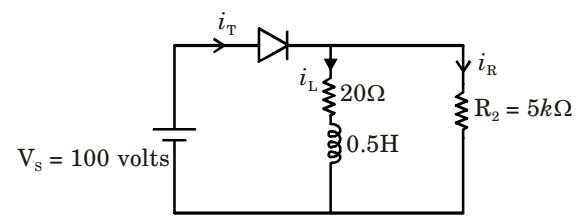
   iR =	100	=	100	= 0.02 A
   	R2		5 × 103

   
   

   =	100		1 - e{-20t / 0.5}		= 5(1 - e-40t)
   	20

   
   Then, i_T = i_L + i_R = 0.02 + 5(1 – e ^{– 40t})
   It minimum pulse width is T for i_a ≥ latching current.
   Then, 0.02 + 5(1 – e ^{– 40t}) = 0.05
   ⇒ 5(1 – e ^{– 0.40t}) = 0.03
   ⇒ t = 150 μ sec.

   Correct Option: A

   
   

   iR =	100	=	100	= 0.02 A
   	R2		5 × 103

   
   

   =	100		1 - e{-20t / 0.5}		= 5(1 - e-40t)
   	20

   
   Then, i_T = i_L + i_R = 0.02 + 5(1 – e ^{– 40t})
   It minimum pulse width is T for i_a ≥ latching current.
   Then, 0.02 + 5(1 – e ^{– 40t}) = 0.05
   ⇒ 5(1 – e ^{– 0.40t}) = 0.03
   ⇒ t = 150 μ sec.

---

27. In the given rectifier, the delay angle of the thyristor T₁ measured from the positive going zero crossing of V_s is 30°. If the input voltage V_s is 100 sin(100πt)V, the average voltage across R (in Volt) under steady-state is _____________.
    

1. 1. 11.25

   
   
   

   2. 61.52

   
   
   

   3. 21.52

   
   
   

   4. 71.25

   
   
   

---

1. View Hint View Answer Discuss in Forum

   Given : α = 30° ,
   V_in = 100 sin(l00πt)
   

   Now , V0 =	Vm	[3 + cosα]
   	2π

   
   

   =	100	[3 + cos30°] = 61.52 V
   	2π

   Correct Option: B

   Given : α = 30° ,
   V_in = 100 sin(l00πt)
   

   Now , V0 =	Vm	[3 + cosα]
   	2π

   
   

   =	100	[3 + cos30°] = 61.52 V
   	2π

---

---

28. In the following chopper duty ratio of switch S is 0.4. If the inductor and capacitor are sufficiently large to ensure continuous inductor current and ripple free capacitor voltage, the charging current (in Ampere) of the 5V battery, under steady-state, is _____________ .
    
    

1. 1. 1

   
   
   

   2. 2

   
   
   

   3. 3

   
   
   

   4. 4

   
   
   

---

1. View Hint View Answer Discuss in Forum

   V₀ = DV_S = 0.4 × 20 = 8 V
   

   I0 =	V0 - E	=	8 - 5	=	3	= 1 A
   	R		3		3

   Correct Option: A

   V₀ = DV_S = 0.4 × 20 = 8 V
   

   I0 =	V0 - E	=	8 - 5	=	3	= 1 A
   	R		3		3

---

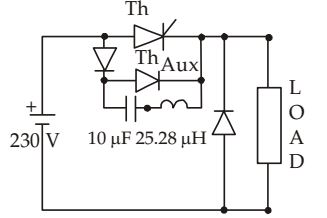
29. The circuit in the figure is a current commutated dc – dc chopper where, Th_M is the main SCR and Th_AUX is the auxiliary SCR. The load current is constant at 10 A. Th_M is ON. Th_AUX is triggered at t = 0. Th_M is turned OFF between
    
    

1. 1. 0 μs < t ≤ 25 μs

   
   
   

   2. 25 μs < t ≤ 50 μs

   
   
   

   3. 50 μs < t ≤ 75 μs

   
   
   

   4. 75 μs < t ≤ 100 μs

   
   
   

---

1. View Hint View Answer Discuss in Forum

   
   For t < 0, V_c = V_s, i_c = 0 and i_T1 = I₀
   At t = 0, TH_AUX is trigered, and a resonant Current i c designs to flow from C through TH_AUX, L and back to c.
   The resonant current is given by,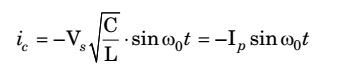
   
   

   At t1 =	π	, ic = 0 and Vc = -Vs
   	ω0

   
   Since i_C gets reverses, TH_AUX is off.
   For V_c = – V_s , resonant current i_c flows through C, L, D and TH_m. Because this current i_c flows and builds up in opposite direction to forward current of TH_m Then,
   i_m = I_o – i_c = I_o – I_p sin ω∆t
   when i_m = 0, TH_m gets turned off
   

   i.e. , ∆t =	1	sin-1		Io
   	ω0			Ip

   
   Hence, TH_m is off between t₁ < t < t₁ + ∆t
   

   t1 =	π	= π√LC = π√10 × 25.28 sec = 50 sec.
   	ω0

   
   i.e., commutation time given by 50 µs < t < 75s

   Correct Option: B

   
   For t < 0, V_c = V_s, i_c = 0 and i_T1 = I₀
   At t = 0, TH_AUX is trigered, and a resonant Current i c designs to flow from C through TH_AUX, L and back to c.
   The resonant current is given by,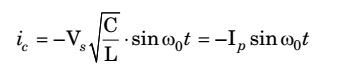
   
   

   At t1 =	π	, ic = 0 and Vc = -Vs
   	ω0

   
   Since i_C gets reverses, TH_AUX is off.
   For V_c = – V_s , resonant current i_c flows through C, L, D and TH_m. Because this current i_c flows and builds up in opposite direction to forward current of TH_m Then,
   i_m = I_o – i_c = I_o – I_p sin ω∆t
   when i_m = 0, TH_m gets turned off
   

   i.e. , ∆t =	1	sin-1		Io
   	ω0			Ip

   
   Hence, TH_m is off between t₁ < t < t₁ + ∆t
   

   t1 =	π	= π√LC = π√10 × 25.28 sec = 50 sec.
   	ω0

   
   i.e., commutation time given by 50 µs < t < 75s

---

---

30. A single-phase bridge converter is used to charge a battery of 200 V having an internal resistance of 0.2 Ω as shown in the figure given below. The SCRs are triggered by a constant dc signal. If SCR 2 gets open circuited, then what will be the average charging current?
    
    

1. 1. 23.8 A

   
   
   

   2. 15 A

   
   
   

   3. 11.9 A

   
   
   

   4. 3.54 A

   
   
   

---

1. View Hint View Answer Discuss in Forum

   Average current is given by,
   
   

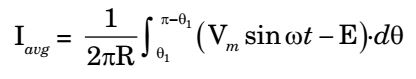
   =	1	[2Vm cosθ - E(π - 2θ1)]
   	2πR

   
   

   where , θ1 = sin-1			E
   			Vm

   
   

   = sin-1			200		= 38° = 6.66 rad
   			230 √2

   
   

   Then , Iavg =	1
   	2π × 2

   
   

   Iavg =	1	[2 √2 × 230 cos38° - 200(π - 2 × 0.66)] = 11.9
   	2π × 2

   Correct Option: A

   Average current is given by,
   
   

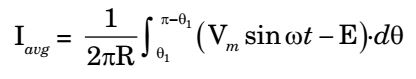
   =	1	[2Vm cosθ - E(π - 2θ1)]
   	2πR

   
   

   where , θ1 = sin-1			E
   			Vm

   
   

   = sin-1			200		= 38° = 6.66 rad
   			230 √2

   
   

   Then , Iavg =	1
   	2π × 2

   
   

   Iavg =	1	[2 √2 × 230 cos38° - 200(π - 2 × 0.66)] = 11.9
   	2π × 2

---