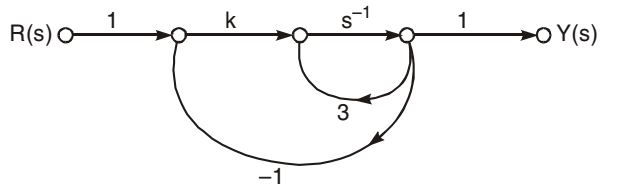
51. The system shown in the figure remains stable when
    
    

1. 1. K < – 1

   
   
   

   2. – 1 < K < 1

   
   
   

   3. 1 < K < 3

   
   
   

   4. K > 3

   
   
   

---

1. View Hint View Answer Discuss in Forum

   	Y(s)	=	K
   	R(s)		s - 3 + K

   
   The system will stable, when single pole lies in the RH-s plane, i.e.
   K – 3 > 0
   ⇒ K > 3

   Correct Option: D

   	Y(s)	=	K
   	R(s)		s - 3 + K

   
   The system will stable, when single pole lies in the RH-s plane, i.e.
   K – 3 > 0
   ⇒ K > 3

---

52. None of the poles of a linear control system lie in the right half of s-plane. For a bounded input, the output of this system
    

1. 1. is always bounded

   
   
   

   2. could be unbounded

   
   
   

   3. always tends to zero

   
   
   

   4. none of these

   
   
   

---

1. View Hint View Answer Discuss in Forum

   For a linear control system with no poles in R.H.S. of s-plane including roots on jω axis with bounded input, the output may be unbounded.

   Correct Option: B

   For a linear control system with no poles in R.H.S. of s-plane including roots on jω axis with bounded input, the output may be unbounded.

---

---

53. An electromechanical closed-loop control system has the following characteristic equation :
    s³ + 6 Ks² + (K + 2) s + 8 = 0
    where K is the forward gain of the system.
    The condition for closed loop stability is
    

1. 1. K = 0.528

   
   
   

   2. K = 2

   
   
   

   3. K = 0

   
   
   

   4. K = – 2.58

   
   
   

---

1. View Hint View Answer Discuss in Forum

   s³ + 6 Ks² + (K + 2)s + 8 = 0
   
   

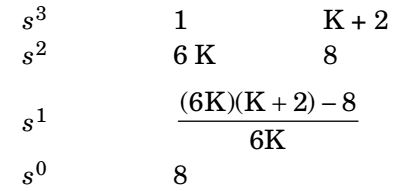
   For stablility,	(6K)(K + 2) - 8	= 0
   	6

   
   ⇒ K > 0
   ∴ 3 K² + 6 K – 4 > 0
   

   ⇒ K =	-6 ± √36 + 48
   	6

   
   

   =	-6 ± √84	= 0.528 , -2.58
   	6

   
   Since K > 0, hence K = 0.528
   

   Correct Option: A

   s³ + 6 Ks² + (K + 2)s + 8 = 0
   
   

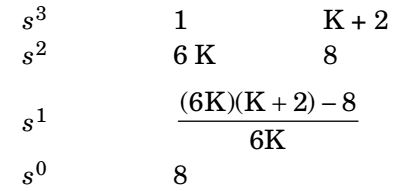
   For stablility,	(6K)(K + 2) - 8	= 0
   	6

   
   ⇒ K > 0
   ∴ 3 K² + 6 K – 4 > 0
   

   ⇒ K =	-6 ± √36 + 48
   	6

   
   

   =	-6 ± √84	= 0.528 , -2.58
   	6

   
   Since K > 0, hence K = 0.528
   

---

54. The number of roots of s³ + 5 s² + 7s + 3 = 0 in the right half of the s-plane is
    

1. 1. zero

   
   
   

   2. one
      

   
   
   

   3. two

   
   
   

   4. three

   
   
   

---

1. View Hint View Answer Discuss in Forum

   Number of roots of s³ + 5s² + 7s + 3 = 0 in the LHS of s-plane
   
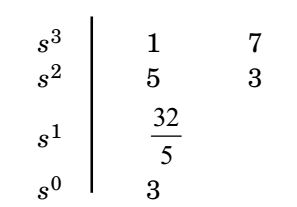
   Since no sign change, hence no root in RHS of splane.

   Correct Option: A

   Number of roots of s³ + 5s² + 7s + 3 = 0 in the LHS of s-plane
   
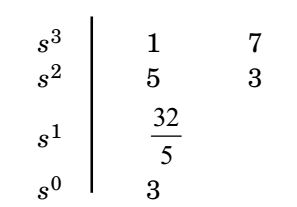
   Since no sign change, hence no root in RHS of splane.

---

---

55. The Nyquist plot for the open-loop transfer fucntion G(s) of a unity negative feedback system is shown in the figure. If G(s) has no pole in the right-half of s-plane, the number of roots of the system characteristic equation in the right-half of s-plane is
    
    

1. 1. 0
      

   
   
   

   2. 1
      

   
   
   

   3. 2
      

   
   
   

   4. 3

   
   
   

---

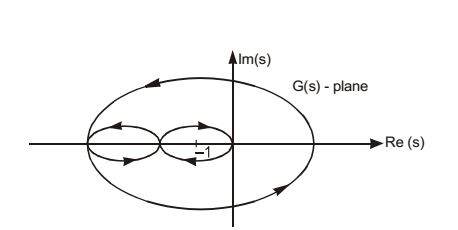
1. View Hint View Answer Discuss in Forum

   P = 0
   N = 0, since encirclement is zero,
   As, P = N + Z.
   ∴ Z = 0.

   Correct Option: A

   P = 0
   N = 0, since encirclement is zero,
   As, P = N + Z.
   ∴ Z = 0.

---