Thermodynamics Miscellaneous
Direction: Air enters an adiabatic nozzle at 300 kPa, 500 K with a velocity of 10 m/s. It leaves the nozzle at 100 kPa with a velocity of 180 m/s. The inlet area is 80 cm². The specific heat of air c is 1008 J/kgK
- The exit temperature of the air is
-
View Hint View Answer Discuss in Forum
h1 = V²1 = h2 + V²2 2 2 ⇒ V²2 - V²1 = h1 - h2 = Cρ(T1 - T2) 2 &There4; 180² - 10² = 1008 × (500 - T2) 2
⇒ T2 = 484KCorrect Option: C
h1 = V²1 = h2 + V²2 2 2 ⇒ V²2 - V²1 = h1 - h2 = Cρ(T1 - T2) 2 &There4; 180² - 10² = 1008 × (500 - T2) 2
⇒ T2 = 484K
- Steam enters a well insulated turbine and expands isentropically throughout. At an intermediate pressure, 20 percent of the mass is extracted for process heating and the remaining steam expands isentropically to 9 kPa.
Inlet to turbine: P = 14 MPa, T = 560°C,
h = 3486 kJ/kg, s = 6.6 kJ/(kgK)
Intermediate stage: h = 276 kJ/kg
Exit of turbine: P = 9 kPa, hf = 174 kJ/kg,
hg = 2574 kJ/kg, sf = 0.6 kJ/(kgK), sg = 8.1 kJ/(kgK)
If the flow rate of steam entering the turbine is 100 kg/s, then the work output (in MW) is____.
-
View Hint View Answer Discuss in Forum
h1 = 3486 kJ/kg
h2 = 2776 kJ/kg
s1 = s3 = 6.6
6.6 = .6 + x(0.1 – .6)
x = 0.8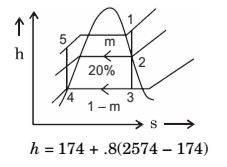
= 2094 kJ/kg
w = (3486 – 2776) + .8 (2776 – 2094)
= 1255.6 kJ/kg = 125.56 MWCorrect Option: B
h1 = 3486 kJ/kg
h2 = 2776 kJ/kg
s1 = s3 = 6.6
6.6 = .6 + x(0.1 – .6)
x = 0.8
= 2094 kJ/kg
w = (3486 – 2776) + .8 (2776 – 2094)
= 1255.6 kJ/kg = 125.56 MW
- The mass flow rate of air through the nozzle in kg/s is
-
View Hint View Answer Discuss in Forum
Mass flow rate, m = ρQ
where, Q = A2 V2
But V2 = √2CP(T1 - T2)
= √2 × 1.005 × (400 - 239.5) = 568 m/s
∴ m = 0.727 × 0.005 × 568 = 2.06 kg/sCorrect Option: D
Mass flow rate, m = ρQ
where, Q = A2 V2
But V2 = √2CP(T1 - T2)
= √2 × 1.005 × (400 - 239.5) = 568 m/s
∴ m = 0.727 × 0.005 × 568 = 2.06 kg/s
- The thermal efficiency of an air-standard Brayton cycle in terms of pressure ratio rρ and γ(= cρ /cv) is given by
-
View Hint View Answer Discuss in Forum
Thermal efficiency of air standard efficiency = 1 – 1 (rρ)γ-1/γ Correct Option: D
Thermal efficiency of air standard efficiency = 1 – 1 (rρ)γ-1/γ
- The exit area of the nozzle in cm² is
-
View Hint View Answer Discuss in Forum
From continuity equation, we get
ρ1 A1 V1 = ρ2 A2 V2∴ P1 × A1V1 = P2 × A2V2 RT1 RT2 ⇒ A2 = P1T2 × V2 × A1 300 × 484 × 10 × 80 = 12.9 cm² P2T1 V2 500 × 100 180 Correct Option: D
From continuity equation, we get
ρ1 A1 V1 = ρ2 A2 V2∴ P1 × A1V1 = P2 × A2V2 RT1 RT2 ⇒ A2 = P1T2 × V2 × A1 300 × 484 × 10 × 80 = 12.9 cm² P2T1 V2 500 × 100 180

