Direction: A cylindrical container of radius R = 1 m, wall thickness 1 mm is filled with water up to a depth of 2 m and suspended along its upper rim. The density of water is 1000 kg/m3 and acceleration due to gravity is 10 m/s2. The self-weight of the cylinder is negligible. The formula for hoop stress in a thin-walled cylinder can be used at all points along the height of the cylindrical container.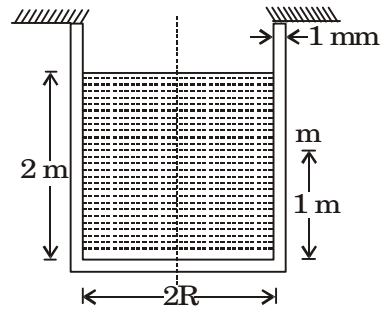
-
If the Young's modulus and Poisson's ratio of the container material' are 100 GPa and 0.3, respectively, the axial strain in the cylinder wall at mid-depth is
-
- 2 × 10–5
- 6 × 10–5
- 7 × 10–5
- 1.2 × 10–4
Correct Option: A
Axial strain in the cylinder would be produced due to the axial (longitudinal) stress and due to the lateral stress
| i.e.hoop stress, e = |  | σu - |  | |||
| y | m |
| = | (5 - 10 × 0.3) | 100 × 103 |

