-
From an aeroplane just over a straight road, the angles of depression of two consecutive kilometre stones situated at opposite sides of the aeroplane were found to be 60° and 30° respectively. The height (in km) of the aeroplane from the road at that instant was (Given √3 = 1.732)
-
- 0.433
- 8.66
- 4.33
- 0.866
Correct Option: D
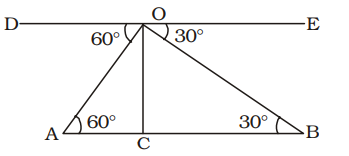
OC = Height of plane = h km (let)
∠DOA = ∠OAC = 60° ;
∠BOE = ∠OBC = 30°
AB = 2 km.
AC = x km (let)
∴ BC = (2 – x ) km.
From, ∆OAC
| tan 60° = | AC |
| ⇒ √3 = | x |
| ⇒ x = = | km. ...(i) | √3 |
From ∆OBC,
| tan 30° = | CB |
| ⇒ | = | √3 | 2 - x |
| ⇒√3 h = 2 – | [From equation(i)] | √3 |
[From equation(i)]
| ⇒√3 h + | = 2 | √3 |
| ⇒ | = 2 | √3 |
⇒ 4h = 2 √3
| ⇒ h = | = | km. | 4 | 2 |
| = | = 0.866 km. | 2 |

